Stress distribution in welds
Once again about how to read the results of weld-check.
One more time about utilisation factor 98% for welds.
Situations where it is not critical and where it is.
And how to use IDEA StatiCa to examine all these effects, of course.
transverse direction
As usual, I will start from an example
Excessively stiff column so that it won’t disturb results.
A small rib of a cross section of 200x10 mm.
Axial tension— 200 kN.
Double weld of 5 mm (throat) of grade S355.
Before we start the CBFEM-calculation, let’s perform a small manual precheck.
Hand calculation to predict the capacity.
Calculation gives the result of a total resistance of two welds of 590 kN.
So the welds utilization factor is expected to be around 0.34, right?
And here we are!
This is probably the most frequently asked question about welds in IDEA StatiCa.
You probably already know the answer, but in any case — look at the next example.
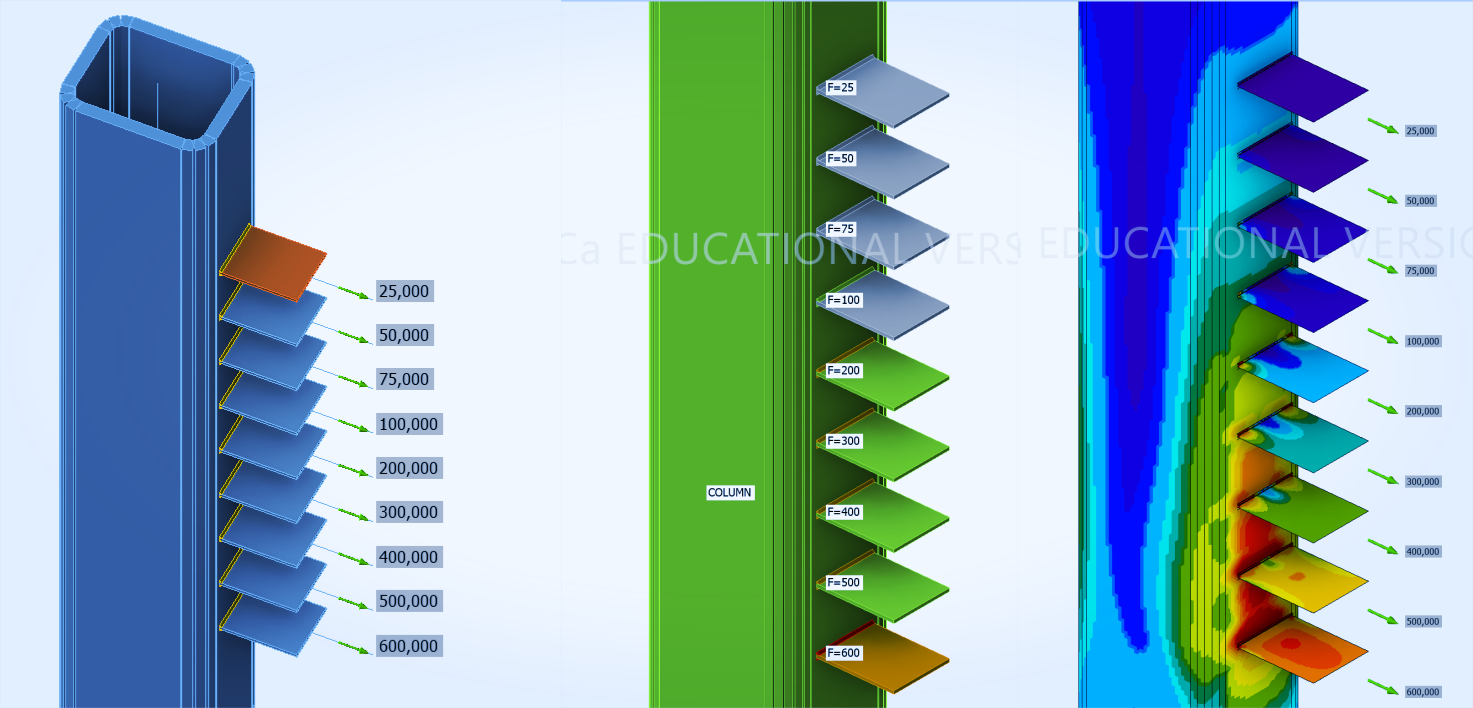
Let’s clone this plate and load it with several different loads at once to see the gradient of results (don’t do it for real joints please!)
So, as we see, plates loaded with 200, 300, 400 and 500 kN — they all have that “orange” welds, that is, the utilization factor is close to 1.0, but doesn’t exceed it.
And only weld with load of 600 kN colored in red, which perfectly corresponds to our manual calculation above.
Here in the pictures below we can see the diagrams of stresses in welds. Each picture represents its own plate, so if you’ll look at them from left to right you can see how stresses are distributed initially and how they change.
At first — why is stress distribution uneven from the very beginning?
The answer is stiffness. Main column is huge, stiff, but its stiffness is not distributed evenly, so weld “feels” it and follows it.
When the rib pulls the column, edges response with much greater stiffness than middle part, and therefore stresses strive to flow to edges.
Stress always tries to choose the “shortest” path— the greater is stiffness the higher is stress level.
How stresses grow
First three pictures may look the same, but numbers differ. Here, on elastic stage, stresses grows almost linearly and they won’t redistribute until some plastic deformations occurs.
Then plasticity comes. Around 200 kN of applied force. Since edge zones initially had a higher stress level, these zones reached plasticity first. When some part of the weld reached the plastic plateau, it rests and stresses no longer increase. This is plastic redistribution.
Here IDEA Statica shows you utilization factor close to 1.0, but it doesn’t mean that connection is about to fail.
The only conclusion you can do — is that connection hasn’t failed yet under exact certain load. This is the thing that is important to understand — utilization factor doesn’t tell you about “allowable stresses” or “how much capacity my connection has”.
But you can always increase load to see the actual load-bearing capacity. And that is what we did. We increased load from 200 kN to 300, then to 400, to 500 and we still had the same utilization factor and pretty same stress level, but the proportion of plastic part of the weld to the elastic part have been grown higher and higher. And only when the whole weld reached plasticity stage, it failed (approximately 600 kN).
On the graph I’ve plot the maximum stresses and applied force for each case. It is bilinear diagram and it is pretty obvious that we cannot judge the joint load-bearing capacity when we stay on plastic plateau. And right here this plateau occupies 2/3 of the whole diagram from zero to weld capacity limit. Thus the chance to see “98%” for weld is really high, so don’t panic when you see it.
welds ductility is not infinite
Unfortunately, plastic deformations can not last forever, and weld stresses diagram will not always flatten itself uniformly.
Let’s create another model, this time something simple.
For example — SHS 160x8 with 120x8 mm plate.
Two welds of 5 mm throat, the same as previously.
Estimated bearing capacity of welds is 354 kN.
354 kN…
But the weld cannot even stand against less than a half of it. After applying only 150 kN, we get the model that is too red.
And 100 kN looks like something very close to limit.
The middle part of the weld doesn’t even try to help! Neither if we apply 100 kN, nor if 150 kN.
This is what in codes is called effective width of weld.
In this particular case we can use formula from EN 1993-1-8
(or CIDECT Design Guide 3).
Formula says that effective part of the weld is only 40 mm or 1/3.
Now look at weld stresses diagrams above. You can measure from the picture that no-working part is exactly 2/3 of the weld.
Whatever happens, in this connection middle part of the weld will not start working.
This more or less trivial cases are covered with known formulas for effective width, thanks to books and codes, but IDEA StatiCa gives you much more flexibility to inspect this effect.
longtitudinal direction
In longitudinal welds highest stresses are situated in the beginning and in the end.
They plastically redistribute in a similar manner.
And the same as for transversal welds, we may be trapped in situation when some part of the weld cannot manage to reach plasticity phase.
Such welds can be compared to what is written for “long joints” in Eurocode 1993-1-8.
Practical example
Okay, we saw the weld ductility in some “laboratory” tests above. How will we use this knowledge for solving practical problems?
Here we have and end-plate splice under axial tension.
Ribs have 90 mm width and 220 mm depth.
We can see that all welds to endplate are “red”
Such connections can be very sensitive to welding because double welds are not possible for SHS and welds here are heavyloaded.
Let’s try to “expand” the connection and, therefore, ribs-to-endplate welds length
Utilization factor is only 105%.
There are almost half of all welds length on ribs. So it looks like we need just +10% to ribs width, right?
So 90 mm → 120 mm should be enough. Or not? :)
Ribs width = 120 mm. We have 16% more welds length.
But utilization is still over 1.0.
Let’s continue.
Ribs width = 150 mm!
133% of weld length!
Hand calculation can say that utilization factor should be proportional to welds length in such a simple problem, but it is not!
Finally, it is time to examine how plastic processes work for our welds.
Here it is!
“Plastic strain” results mode gives us the glimpse of the answer.
The furthest part of rib is too far from where the stiffness is concentrated. It is away from the main element, away from the bolt. So its help is unequal.
Moreover, we increased the width of ribs, but didn’t extend their depth, so stresses can not reach the furthest parts of ribs.
Of course we can solve this problem by increasing welds throat or by moving bolts a little or by changing ribs arrangement and quantity.
But I just wanted to demonstrate an example where uneven distribution of stresses in the weld gives a difference. The more complicated your joint is, the more complex is the load combination — the less the less likely it is to achieve ideal, uniform distribution.
Bending moments
You are probably thinking
“Plastic redistribution for longitudinal and transverse forces are more-or-less clear, but how about the bending moment?”
Obviously, some plasticity on the edges is possible. But if your weld is not full-strength fillet or butt weld, I would not recommend to design such welds with a lot of plastic deformation.
And even if your weld is full-strength, please, don’t expect it to turn into a working plastic hinge:
Conclusion
Welds have the property of ductility. This ductility gives them the ability to redistribute stresses
Ductility is not infinite and in some cases part of the weld stays inactive even when other part fails
Utilization factor of 98% doesn’t always mean that we are close to safety margin.
Stress uneven distribution can lead to the need for very careful analysis.
PS: “My welds stresses diagrams are not so smooth”
Each “column” on the stresses diagram on a weld in IDEA StatiCa represents one finite element of the model. The more FE you have in your weld the more columns you will see on the diagram.
Here, for the images above, I changed the meshing parameters to make a lot of finite elements for every weld. In practical engineering problems this will most likely bring nothing but headache due to long calculation time. But your calculation is your calculation, you can always choose the mesh parameters that you think suit best.
Still have questions?
If you have any additional questions or want to deepen your knowledge, consider joining one of our comprehensive IDEA StatiCa courses.
Explore our courses to find the one that suits your needs best or request a custom course. Get in touch with us today to learn more or to sign up!